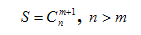UDC 524.8
Ignatyev Mikhail Borisovich – Saint Petersburg State University of Aerospace Instrumentation, professor, International Institute of Cybernetics and Artonics, director, Saint Petersburg, Russia.
E-mail: ignatmb@mail.ru
67, Bolshaya Morskaya, Saint Petersburg, Russia, 190000,
tel: +7(812)494-70-44
Abstract
Background: Galaxies are complex systems, which, in the course of their development, pass a number of cycles, with the periods of adaption maximum being included. The nature of the hypothetical block controlling these systems development is still unknown.
Results: Astrophysical structures – galaxies, star clusters, etc. – are complex cyberphysical systems with a large variety of elements. In the course of their development these astrophysical structures interact with the environment consisting of some other galaxies and larger structures. In addition, the astrophysical structures are under the influence of external and internal control, which is realized through a hypothetical control unit. The arbitrary coefficient manipulation in the structure of equivalent equation, the imposition and lifting of restrictions on system variables, the merging of the systems into a collective one, etc. are considered to be their management tool, which eventually forms the life cycles of galaxies. Astrophysical structures are complex self-organizing systems, so they are subject to all identified patterns of complex systems development.
Conclusion: The existence of adaptation maximum in astrophysical structures life cycle furnishes us with the proposal that they are changed under the influence of highly developed civilizations. If our world is a model within some hypothetical global computer, the study of its system of programming and protection is the essential condition of establishing a contact with them.
Keywords: astrophysical structures; galaxy; stars; black holes; structured uncertainty; the phenomenon of adaptation maximum; external and internal management; life cycle development.
Introduction
The universe consists of many galaxies which are its main elements. The galaxy, in its turn, contains stars and star clusters, black holes and quasars, gravitational and electromagnetic energy, interstellar dust, dark energy and dark matter, and others. Galaxies are studied intensively by means of astrophysics and astronomy. But, on the other hand, galaxies are complex self-organizing systems and they obey the laws of these systems, which is the subject of this article.
1. Linguo-combinatorial modeling
Only a small number of real systems have mathematical models. First of all, the systems are described by using natural language. A method of transition from natural language descriptions to mathematical equations is proposed. For example, suppose there is a phrase:
WORD1 + WORD2 + WORD3 (1)
In this phrase we denote words while the meaning of these words is only implicated. The sense in the current structure of natural language is not indicated. It is proposed to introduce the concept of meaning in the following form:
(WORD1)*(SENSE1)+(WORD2)*(SENSE2)+(WORD3)*(SENSE3)=0 (2)
We denote the word as Ai (Appearance) and the meaning Ei (Essence). Then the equation (2) can be represented as:
A1*E1 + A2*E2 + A3*E3 = 0 (3)
Equations (2) and (3) are phrase models (1). Linguistic and combinatorial model is an algebraic ring, and we can solve the equation (3) either with respect to Ai, or with respect to Ei by introducing a third group of variables, i. e. arbitrary factors Us [2; 5; 6,]:
A1 = U1*E2 + U2*E3
A2 = –U1*E1 + U3*E3 (4)
A3 = –U2*E1 – U3*E2
or
E1 = U1*A2 + U2*A3
E2 = –U1*A1 + U3*A3 (5)
E3 = –U2*A1 – U3*A2,
where U1, U2, U3 – arbitrary coefficients that can be used to solve various problems of diversity (3). In general, if we have n variables and m manifolds, constraints, the number of arbitrary coefficients S will be equal to the number of combinations of n by m+1, that is shown in [2; 5; 6], Table 1:
The number of arbitrary coefficients is a measure of uncertainty and adaptability. Linguistic and combinatorial modeling can be built on the analysis of the entire corpus of natural language texts, this being a time-consuming task of making sense for supercomputers. It can also be used on the basis of the keywords in a specific area, which allows you to obtain new models for specific areas of knowledge. In this case, the combinatorial linguistic modeling is that in specific domain the keywords that are combined in phrases such as (1) are highlighted, for inducing equivalent systems of equations with arbitrary coefficients. In the particular case they may be differential equations, and for their study a well-developed mathematical apparatus can be used. Linguistic and combinatorial simulation includes all combinations and all versions of solutions and is a useful heuristic device in the study of poorly formalized systems [2; 5; 6]. In linguistic literature there are many works, which are exploring the notion of meaning and sense, but these theories are largely proved to be unhelpful, with Ludwig Wittgenstein having shown that clearly in his Blue Book. Using phrase (1) of equation (2) as a model allows you to construct a calculus of meaning, which is well implemented on computers. According to D. A. Leontiev, meaning (whether the meaning of texts, parts of the world, images of consciousness, psychic phenomena, or action) is determined, firstly, through a wider context and, secondly, by intention or entelechy (target orientation, purpose or direction of movement). In our definition of meaning these two characteristics are present, namely contextual (meanings are calculated on the basis of the context) and intentional (arbitrary coefficients allow you to specify certain aspirations) ones.
Table 1
| n /m | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| 2 | 1 | |||||||
| 3 | 3 | 1 | ||||||
| 4 | 6 | 4 | 1 | |||||
| 5 | 10 | 10 | 5 | 1 | ||||
| 6 | 15 | 20 | 15 | 6 | 1 | |||
| 7 | 21 | 35 | 35 | 21 | 7 | 1 | ||
| 8 | 28 | 56 | 70 | 56 | 28 | 8 | 1 | |
| 9 | 36 | 84 | 126 | 126 | 84 | 36 | 9 | 1 |
2. Adaptation possibilities of complex systems
In the structure of the equivalent equation systems with structured uncertainty there are some arbitrary coefficients that can be used to adapt the system to various changes in order to improve the accuracy and reliability of the systems, their survivability in the flow of change. If we take galaxy stars, galaxy quasars, black galaxy holes, gravitational galaxy energy, electromagnetic galaxy energy, dark galaxy energy, dark galaxy matter as the keywords that characterize the galaxy, the galaxy linguistic equation in accordance with the procedure mentioned above will be
А1*Е1 + А2*Е2 + … + А7*Е7 = 0, (7)
and equivalent equations will have the following form:
E1 = U1*A2 + U2*A3 + U3*A4 + U4*A5 + U5*A6 + U6*A7;
E2 = –U1*A1 + U7*A3 + U8*A4 + U9*A5 + U10*A6 + U11*A7;
E3 = –U2*A1 – U7*A2 + U12*A4 + U13*A5 + U14*A6 + U15*A7;
E4 = –U3*A1 – U8*A2 – U12*A3 + U16*A5 + U17*A6 + U18*A7; (8)
E5 = –U4*A1 – U9*A2 – U13*A3 – U16*A4 + U19*A6 + U20*A7;
E6 = –U5*A1 – U10*A2 – U14*A3 – U17*A4 – U19*A5 + U21*A7;
E7 = –U6*A1 – U11*A2 – U15*A3 – U18*A4 – U20*A5 – U21*A6,
where A1 – the characteristic of stellar galaxy population; E1 – this characteristic change; A2 – the characteristic of quasar galaxy population; E2 – this characteristic change; A3 – the characteristic of black galaxy holes, E3 – this characteristic change; A4 – the characteristic of gravitational galaxy energy; E4 – this characteristic change; A5 – the characteristic of electromagnetic galaxy energy; E5 – this characteristic change; A6 – the characteristic of dark galaxy energy; E6 – this characteristic change A7 – the characteristic of dark galaxy matter; E7 – this characteristic change; U1, U2, …, U21 – arbitrary coefficients. The number of keywords and the number of restrictions such as (7) can change, but the structure is equivalent to the equation of type (8), the number of arbitrary factors and their distribution in the matrix of these equations will change. For example, if the keywords of the galaxy include nine words [1] – diameter D25, drive radial scale R0, the thickness of the stellar disk, luminosity, mass M25 within D25, the relative weight of the gas within D25, the rotational speed of the outer regions of the galaxy, the period of revolution the outer regions of the galaxy, the mass of the central black hole, the structure of the equivalent equations will contain 36 arbitrary coefficients.
Figure 1 shows the communication structure of the system – in this case the galaxy – with the environment. The result of this interaction is the occurrence of delta signals, which affect both the system and the environment. The system has a hypothetical control unit that acts on the body while manipulating arbitrary coefficients, applying and removing restrictions, etc.
Figure 1. The communication structure of the system (the galaxy) with the environment.
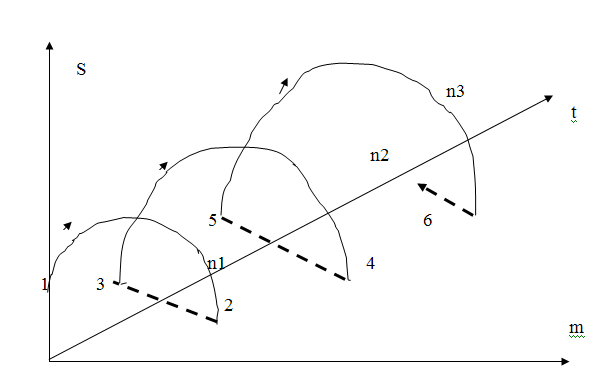
As a result of interaction with the environment, the galaxy evolves as shown in Figure 2.
Figure 2. Transformation of developing galaxies, n1 <n2 <n3, the trajectory of the system: … 1-2-3-4-5-6-, the solid line shows the evolutionary processes, the broken line shows the creative processes.
As part of the linguistic and combinatorial approach, complex systems are described by equivalent equations with arbitrary coefficients, the matrix of which depends on the number of variables and the number of restrictions. The number of arbitrary coefficients is defined as the number of combinations of n by m+1, where n – the number of different elements of the galaxy, m – the number of constraints imposed on them. The number of arbitrary factors characterizes the adaptive capacity of the galactic system. In the course of evolution, the galaxy passes through a maximum adaptation and gradually turns into rigid systems that either die or are transformed with the help of creative transition by lifting accumulated restrictions – see Fig. 2. The cycle of galaxy development begins at 1, passes through its maximum in the number of arbitrary factors and ends at 2, where the transformation, i. e. the removal of the previously accumulated restrictions, has to occur, the new cycle begins at point 3, the system has a maximum capacity of adaptation again, it reaches point 4, where again there is some transformation, etc. Similar cycles are present in biological, social, economic and technical systems [2; 6]. The question arises, what is the nature of a hypothetical control unit in the system in Figure 1? This is the subject of further research and there are several possible options, either it is a special cyber-physical structure similar to automatic systems [2; 6] or some manifestation of life and an advanced civilization.
Conclusion
The evolution of galaxies keeps many secrets, one of which is that the evolution of galaxies is largely determined by the presence of life in the universe.
For hundreds of years people have been trying to meet with aliens, but our space messages remain unanswered. Many scientists – mathematician Carl Gauss, astronomers Carl Sagan and Frank Drake, and many others have tried to solve this problem, a lot of money has been spent, but there is no result. Why is that so?
Because the dominant physicalist picture of the world is probably not perfect, as evidenced by many reports on XXIX General Assembly of the International Astronomical Union (IAU XXIX GA). Indeed, astrophysicists have discovered dark matter and dark energy, which make up 95 % of the mass and energy of the universe, but our view of the world is based on the observations of only 5 % of the mass and energy of the universe. It is believed that dark matter and dark energy are indirect evidence of life in the Universe [3; 6; 8; 9]. In beginning of the XX century V. Vernadskiy formulated his noosphere concept, according to which mankind’s activity is compared to the geological one. Nowadays we have to introduce the noosphere concept into the Universe. On the one hand, the life in the Universe can exert a considerable influence on astrophysical structures [3; 8; 9].
On the other hand, the computer has appeared in which there exist different non-overlapping virtual worlds. This is obvious to everyone who owns a computer. An analogy can be drawn, our world is probably a model within some global supercomputer, where there are many different worlds, and each of them is equipped with a powerful security system from intrusion [4; 6; 7]. If you carry out the research in this field, it is necessary to examine the structure of that global supercomputer, the methods of its programming and protection, and only then you will be able to overcome the protection of the world’s supercomputers and to communicate with other civilizations.
References
1. Sparke L. S., Gallager J. S. Galaxies in the Universe: An Introduction. Cambridge, CambridgeUniversity Press, 2007, 442 p.
2. Ignatyev M. B. Holonomical Automatic Systems. Moscow–Leningrad, Akademiya nauk SSSR, 1963, 204 p.
3. Kardashev N. S. Transmission of Information by Extraterrestrial Civilization. Soviet Astronomy, Vol. 8, № 2, 1964.
4. Ignatyev M. B. Philosophical Problems of Computerization and the Simulation. XXVII Congress of CPSU and Challenges of Improving the Work of Philosophical (Methodological) Seminars, Leningrad, Akademiya nauk SSSR, 1987, 115 p.
5. Ignatyev M. B. Linguo-Combinatorial Simulation in Modern Physics. American Journal of Modern Physics, 2012, Vol. 1, № 1, pp. 7–11. DOI: 10.11648/j.ajmp.20120101.12.
6. Ignatyev M. B. Cybernetic Picture of the World. Complex Cyber-Physical Systems. Saint Petersburg, GUAP, 2014, 472 p.
7. Papakonstantinou Y. Created Computed Universe. Communication of ACM, 2015, Vol. 58, № 6, pp. 36–38. DOI: 10.1145/2667217.
8. Ignatyev M., Parfinenko L. Galaxy Evolution Simulation on Basement of the Linguo-Combinatorial Approach. Proceedings of the International Astronomical Union XXIX General Assembly, Symposium 317, 2015.
9. Ignatyev M., Parfinenko L. Star Clasters Evolution Simulation on Basement of the Linguo-Combinatorial Approach. Proceedings of the International Astronomical Union XXIX General Assembly, Symposium 316, 2015.
Ссылка на статью:
Ignatyev M. B. System Analysis of Astrophysical Structures // Философия и гуманитарные науки в информационном обществе. – 2015. – № 3. – С. 85–91. URL: http://fikio.ru/?p=1798.
© M. B. Ignatyev, 2015